Platonism Essay ⋆ History Essay Examples ⋆ EssayEmpire

Platonism is the philosophy or worldview of Plato, a Greek scholar who believed in a world beyond the everyday world, a world in which things were more real and vital than the world that one typically perceives with one’s senses. Plato, who lived 427–347 b.c.e., was a citizen of Athens. Socrates, who Plato called “the most just man of our times,” taught him. Socrates claimed that he was only wiser than others in that, “I know what I do not know.” Socrates did not write anything down; it is largely through the writings of Plato that modern readers learn about Socrates. Plato attempted to defend Socrates when he was tried and put to death, but the judges were quite biased against Socrates. Following the death of Socrates, Plato traveled the known world in search of further training, studying geometry from Euclid, mystical philosophy from the Italian schools founded by Pythagoras, mathematics from the African Theodorus, and philosophy in Egypt. Eventually, he opened the Academy, outside of Athens, where he taught philosophy. Plato taught Aristotle, who taught Alexander the Great.

Ideas (Forms) And Particular Instances

Central to Plato’s worldview is the reality of archetypal Ideas, often mistranslated as Forms. These Ideas are reflected in our language: A flower is an idea, but that small sunflower that one steps on is a particular instance of a flower. The fact that we have a word for flower indicates that we have an abstract, archetypal concept—an Idea of a flower. Plato says that this idea is more real because unlike the sunflower, which fades and dies, the Idea of a flower lives on. Plato does not say where Ideas are, but modern scholars clearly state that time and space does not apply to Ideas; as above, the Idea of a flower does not die. Though ideas are not seen in the normal way, Plato is convinced that they can be apprehended through the means of intelligence and reason.

Doctrine Of Recollection

In his Meno dialogue Plato has a Socrates character assert that we do not learn things so much as recollect them. The human spirit was trapped in a body and forgot everything but can remember it without outside help. Meno was skeptical of this, and in the dialogue, Socrates answers Meno’s skepticism by calling over an uneducated boy. Socrates clearly demonstrates that the lad lacks all training in geometry. Socrates then sets before the slave a problem involving squares, triangles, and trying to double the size of a given square. Socrates provides no information, but keeps prodding the boy to look at the problem. The boy solves the problem easily and elegantly enough that any reader can follow the steps to the solution. Scholars call this an example of a priori knowledge—knowledge that does not come from prior experience. Plato and his Socrates character assert that all humans have an innate knowledge of geometry from before birth, which can be recollected. Modern mathematics is founded upon this doctrine, that mathematics is part in the world of archetypal Ideas and can be discovered or recalled through mathematical research.

A square is only an Idea of a square, due to imperfections in the thickness of the lines, for example.
The Idea of a square is based on the Idea of a line, the Idea of a right angle, etc.
Since a human cannot see an infinitely thin line, it is assumed that such lines exist. Geometry assumes that the Ideas of squares, lines, and points exist.
Ordinary geometry cannot exist without these basic assumptions.
The assumptions cannot be verified.
If the assumptions are changed, then the entire system of geometry has to change with them.
These Ideas, called fundamental assumptions in geometry, are the most pivotal aspect of this branch of mathematics.

Divided Line

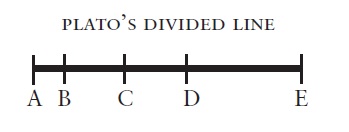Platonism - essay - f1 This concept of a divided line also relates to the Greek notion of the Golden Mean, or Extreme and Mean Ratio. Imagine a line, with points ABCDE.

Let the length of CE be X times longer than the length of AC. Plato declares that AC represents all entities one can comprehend with vision. For instance, a person can see a particular rose, so it is an object in AC. CE represents all things that are comprehensible through intelligence or reason. For example, the Idea of a rose is not something seen with the eyes, but rather something that is apprehended with the heart or mind. CE is longer than AC, and in this diagram, the longer something is, the clearer it is and the easier to comprehend. X is the ratio of the length of CE to the length of AC. This would mean that things apprehended with reason are X times as understandable as those comprehended with mere vision.

Things represented in BC are the ordinary objects.
Things represented in AB are the images of these objects. For example, reflections and shadows are images of objects that cast reflections or shadows.

Plato instructs to make sure that the length of AB is to the length of BC as AC is to CE, or BC/AB = X = CE/ AC. This is an example of the Golden Mean. Images of objects are harder to understand: It is easier to learn to type by looking at the keyboard to see where the keys are, rather than to look at the shadow of the keyboard. Similarly, it is easier to understand all objects by looking at them rather than their images, reflections, or shadows. Now break the line CE into two parts, analogously to the division made in the visible arena:

The lower part, CD, will represent things that are mere images of the things in DE.
Things in CD will be comprehended by understanding, whereas things in DE will be comprehended by reason. And again, the lengths of CD and DE are such that DE/CD = X. In Plato’s terminology, as CD is to DE, so is BC to AB.

Things in CD will be Ideas, like the Idea of a point, the Idea of the line, or the Idea of a square. To get more information about an Ideal square, a geometer draws a picture. The picture is a physical object, seen with vision, so it is in the arena represented by AC, things which are apprehended by sight. Yet one can draw the square on a piece of paper, hold it up to a mirror, and have a reflection of the drawing. Therefore, the drawing is a thing in BC, and the reflected image of the drawing is a thing in AB. This example can explain how to move up the ladder to higher forms of comprehension. The reflection is just an image of the paper, and to better understand the square, one can turn attention not to the reflection, but to the paper on which the square is drawn. And if one goes beyond looking at the drawing of the square to considering the Idea of a square, it is considering a higher form of the concept by turning to the realm of the intelligence.

The reflection is a mere image of the physical object on which the square is drawn, because everything in category AB is a mere image of something in category BC. However, this physical object is a mere image of the Idea of a square. This teaches that just as everything in AB is an image of something in BC, everything in BC is an image of something in CD. Because of how the line is constructed, everything in CD is an image of something in DE, so things in each category are mere images of things in the category above. And just as it is easier to understand something by looking at the object itself than by looking at its image, it is always easier to understand the world by looking at a higher category. Plato claims it is still easier to comprehend the world by looking at the higher-level ideas in DE than the lower-level ideas in CD. The ideas in CD are mere images of the ideas in DE. The higher ideas in DE partake more directly of the Idea of goodness than do the Ideas in CD. The Ideas in CD are apprehended by reason.

The Ideas of points, lines, and squares are assumptions. Therefore, when reason allows humans to see beyond these assumptions in CD to the clearer and more intelligible things above it in DE, they will achieve an understanding that transcends assumptions. The process by which reason allows a vision of the clearest things in DE is the process that Socrates uses in teaching his students: the process of dialectic. Once this amazing state of seeing the thing in DE has been achieved, one can then use this new understanding to move down the line, by first creating a better assumption in CD, and then viewing the consequences of this new assumption, achieving a new and better understanding of the world.

The Allegory Of The Cave

Imagine a group of people born to a cave, where they are chained to stone benches so that they cannot turn around: They are forever facing one large wall of the cave. Behind them is a great bonfire, and between the chained people and the fire, a handful of people hide behind a partition like puppeteers and hold up things to make shadows on the wall at which the others stare. The chained people spend their lives looking at the shadows on the wall and trying to describe them. Thus, the chained people only experience the lowest things mentioned in the divided line discussion—the shadows of objects. All that the chained people know about life comes from their observations of these shadows. The chained people judge one another by their skill at quickly recognizing shadows, and they dislike people who judge poorly or take a long time to recognize the shadows. Plato then describes a process of gradual philosophical awakening. Suppose a chained person breaks free, turns around, and sees both the fire and the people who make the shadows. Plato remarks that his eyes will initially be blinded by the firelight, and the things he sees will appear less real than the shadows he has spent his whole life watching.

But, over time the freed individual’s eyes will adjust to the fire, and he will be able to see it and the puppets that are held up to make the shadows. Perhaps he will realize that what he has been looking at his whole life are not real things but shadows of puppets. Perhaps then the freed prisoner will ascend the long passage that leads from the underground cave to the surface. Imagine that he is compelled to do so quickly. When he arrives at the surface, the light will be too bright and will overwhelm the prisoner’s eyes. At first, the prisoner will see nothing, and then perhaps he will be able to see the shadows of objects that are in the sunlight. In this upper world the shadows are images of the real objects in the sunlight; hence they are like the things represented in CD, in the discussion of the Divided Line. Plato says that in time the freed prisoner may accustom his eyes to see actual objects in the light of day and even to look at the Sun itself, and to see what the Sun is and how it moves across the sky to create the seasons. At this point the freed prisoner can begin to understand what life is and how it works, because he is contemplating the things represented in the category DE from the Divided Line discussion; he is contemplating things that can only be perceived by the true light of reason. At this point the freed prisoner becomes a philosopher. Plato notes that the freed prisoner will desire to remain in the sunlight contemplating the higher things by the light of reason, since the shadows in the cave will seem trivial to him. The newly created philosopher, understanding things by the light of reason will have no desire to discuss shadows.

Yet, Plato asserts that this is exactly what is required for society to improve: The philosopher must return to the cave. No one else understands things as they really are, since everyone else is talking about shadows of puppets, and only the philosopher who understands the nature of the world can lead the people. However, Plato notes that upon returning to the cave, the philosopher will be unaccustomed to the darkness and will at first perform poorly in the shadow-naming contests and be unable even to see the shadows. The prisoners will laugh at the philosopher and think that his journey to the sunlight has ruined his vision. If someone else were to try to free these prisoners by showing them the fire, they would try to kill that person rather than having their vision ruined like they believe the philosopher’s vision has been destroyed. Plato asserts that if the philosopher remains in the cave and becomes reacclimated to the darkness, the philosopher might be able to get others to the surface most quickly, and the philosopher might teach them to see in the shortest period of time. That art is the dialectic study of philosophy, which is how Socrates taught Plato and others.

References:

Hamilton, Edith, and Huntington Cairns, eds. Plato: Collected Dialogues. Princeton, NJ: Princeton University Press, 1985;
Liddell, H. G., and Robert Scott, eds. An Intermediate Greek English Lexicon. Oxford: Clarendon Press, 1986; Peck, Harry Thurston. Harpers Dictionary of Classical Antiquities. New York: Harper and Brothers, 1898.

This example Platonism Essay is published for educational and informational purposes only. If you need a custom essay or research paper on this topic please use our writing services. EssayEmpire.com offers reliable custom essay writing services that can help you to receive high grades and impress your professors with the quality of each essay or research paper you hand in.