Impossibility Theorem Essay ⋆ Political Science Essay Examples ⋆ EssayEmpire

Society has a constant need to aggregate preferences. On a personal level, when making decisions, an individual has to make a rational choice based on several criteria. Taking a presidential election as an example, when deciding which candidate to vote for, the constituent has to take into consideration the candidate’s previous history, political party, and program, among other factors. On a group level, voting systems are built with the purpose of extracting the decision from different voters’ preferences. In this case, legislatures are the most common example. Legislatures have their own voting rules that are used to translate individual preferences into a group preference.

Conditions Of The Impossibility Theorem

The impossibility theorem states that it is impossible to create a voting system that will guarantee a constant set of preferences for a group corresponding to the preferences of the individuals making up that group and offer more than two reasonable choices to the individual. Kenneth Arrow proposes in Social Choice and Individual Values (1951) that no system could be both rational and egalitarian, and that even in a simple voting system the paradox of voting will arise. The theorem demonstrates that no voting system based on ranked preferences can possibly meet a certain set of reasonable criteria when there are three or more options from which to choose. The criteria or conditions that Arrow mentions include the following:

Rationality assumption. Social (or group) preferences should be (1) “complete” in that given a choice between alternatives A and B, individuals from this group should say whether A is preferred to B or B is preferred to A or that there is a social indifference between A and B and (2) “transitive”; that is, individuals have to be coherent about their preferences—if A is preferred to B and B is preferred to C, then A is also preferred to C.

Universal admissibility assumption. Each individual of a group may adopt any strong or weak complete and transitive preference ordering over the alternatives of a given set of alternatives.

Pareto optimality or unanimity condition. If every individual in the group prefers A to B, then socially A should be preferred to B; in other words, the group preference must reflect that A is preferred to B.

Independence from irrelevant alternatives assumption. If members of the group have their own preferences about alternatives A and B, and these preferences do not change, neither should change the group preference about alternatives A and B. This is true even if individual preferences over other (irrelevant) alternatives in the set of alternatives change. Also, social preferences should be independent of irrelevant alternatives; that is, the social preference of A compared with B should be independent of preferences for other alternatives.

Nondictatorship condition. Social or group preferences should not depend only on or be dictated by the preferences of one individual (i.e., the dictator).

For Arrow, any scheme for producing a group choice that satisfies the first four assumptions described above is either dictatorial or incoherent. In other words, there is a person who drives the group decision, or one—or more—of the group members has intransitive preferences. Shepsle and Bonchek (1997) state that Arrow’s impossibility theorem implies a great trade-off: there is, in social life, a trade-off between social rationality and the concentration of power. Social organizations that concentrate power are more coherent about their decisions; meanwhile, those organizations wherein power is more dispersed are less likely to make coherent decisions.

Using The Impossibility Theorem To Predict Voting Results

Suppose that there are three representatives (X, Y, and Z) in Congress who have to vote for a legislative bill that allocates funds for a social program. The rankings of the three bills (B1, B2, and B3) for each of the representatives are given below. For example, Representative X rates bill 1 (B1) as the number one choice, bill 2 (B2) as the second choice, and bill 3 (B3) as the third choice.

Table 1.

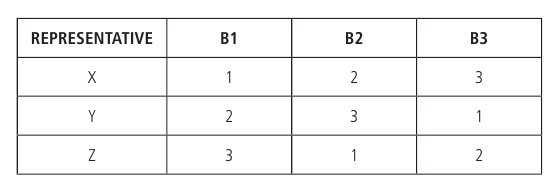
Impossibility Theorem Essay

Another common way to represent the representative’s preferences would be the following:

Representative X: B1 > B2 > B3

Representative Y: B3 > B1 > B2

Representative Z: B2 > B3 > B1

Now let us consider how the vote would go among the three possible pairs of bills. In a vote between two bills, it is assumed that our representatives would vote for the one of the two that is highest in their preferences even though their number one choice may be different from the two being considered.

In a choice between B1 and B2, the X representative would vote for B1 (remember B1 > B2 > B3), the Y representative would also vote for B1 (B3 > B1 > B2), and the Z representative would vote for B2 (B2 > B3 > B1; taking into consideration the transitivity assumption, B2 is preferred to B1). So B1 would win two-thirds of the votes, and we could say that the budget allocation set in B1 is socially preferred to B2.

In a choice between B2 and B3, the X representative would vote for B2 (B1 > B2 > B3), the Y representative would vote for B3 (B3 > B1 > B2), and the Z representative would vote for B2 (B2 > B3 > B1). As a result, B2 would win. So B2 is socially preferred to B3. Rationally, as B1 is preferred to B2 (B1 > B2) and B2 is preferred to B3 (B2 > B3), we would expect that this would imply that B1 would be preferred to B3 (B1 > B3).

However, let us consider a social choice by majority voting between B1 and B3. The X representative would vote for B1 (B1 > B2 > B3), the Y representative would vote for B3 (B3 > B1 > B2), and the Z representative would also vote for B3 (B2> B3 > B1). So B3 is socially preferred to B1. Thus, we have the irrational result that socially, B1 is preferred to B2 and B2 is preferred to B3, but on the contrary, B3 is preferred to B1.

What Arrow was able to prove mathematically is that there is no method for constructing social preferences from arbitrary individual preferences. In other words, there is no rule, majority voting or otherwise, for establishing social preferences from arbitrary individual preferences.

Bibliography:

Arrow, Kenneth Joseph. Social Choice and Individual Values. New York: Wiley, 1951.
Grether, David M., and Charles R. Plott. “Nonbinary Social Choice: An Impossibility Theorem.” Review of Economic Studies 49, no. 1 (1982): 143–149.
Hansen, Paul. Another Simple Graphical Proof of Arrow’s Impossibility Theorem. Dunedin, New Zealand: Department of Economics, University of Otago, 2000.
McKelvey, Richard D., and John Herbert Aldrich. Positive Changes in Political Science:The Legacy of Richard D. McKelvey’s Most Influential Writings. Ann Arbor: University of Michigan Press, 2007.
Poundstone,William. Gaming the Vote:Why Elections Aren’t Fair (and What We Can Do About It). New York: Hill and Wang, 2008.
Schofield, Norman James. The Spatial Model of Politics. London: Routledge, 2008.
Shepsle, Kenneth A., and Mark S. Bonchek. Analyzing Politics: Rationality, Behavior, and Institutions. New York: Norton, 1997.
Tideman, Nicolaus. Collective Decisions and Voting:The Potential for Public Choice. Aldershot, UK: Ashgate, 2006.

This example Impossibility Theorem Essay is published for educational and informational purposes only. If you need a custom essay or research paper on this topic please use our writing services. EssayEmpire.com offers reliable custom essay writing services that can help you to receive high grades and impress your professors with the quality of each essay or research paper you hand in.